Kepler’s Laws of Planetary Motion are three fundamental laws that describe the motion of planets around the Sun. These laws were discovered by the German astronomer Johannes Kepler in the early 17th century. They are a crucial part of our understanding of the cosmos.
In this article, we will delve into each of Kepler’s Laws of Planetary Motion. We will study them in detail so that anyone can understand the beauty and complexity of the laws that govern our solar system.
Kepler’s First Law: The Law of Ellipses
The first of Kepler’s Laws of Planetary Motion is the Law of Ellipses. This law states that the orbit of a planet around the Sun is an ellipse, with the Sun at one of the two foci of the ellipse.

In simpler terms, this means that the path of a planet around the Sun is not a perfect circle. It is an elongated shape that is slightly flattened at the top and bottom. This also means that the distance between the planet and the Sun varies depending on where the planet is in its orbit.
An ellipse is a closed curve that is symmetric about its two axes. One axis is the major axis, and the other is the minor axis. The distance between the two foci is a constant value for any given ellipse, and this distance is known as the major axis.
Kepler’s Second Law: The Law of Equal Areas
The second of Kepler’s Laws of Planetary Motion is the Law of Equal Areas. This law states that a line that connects a planet to the Sun sweeps out equal areas in equal times.
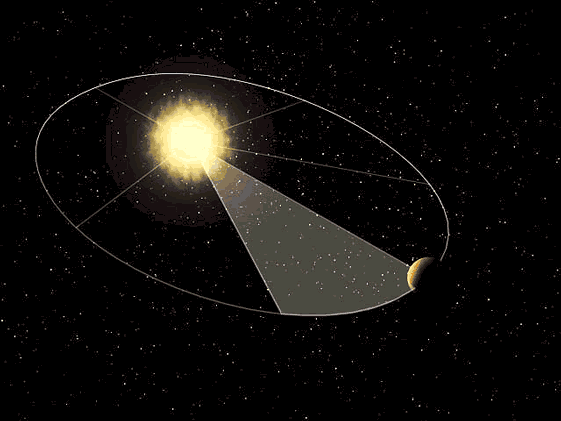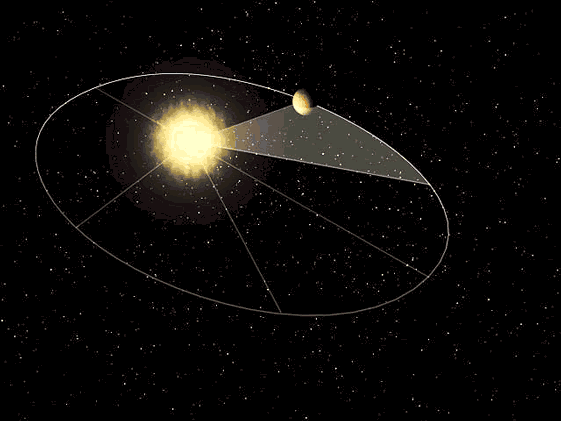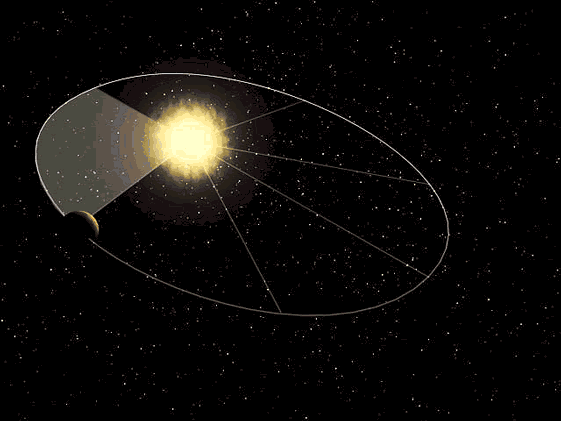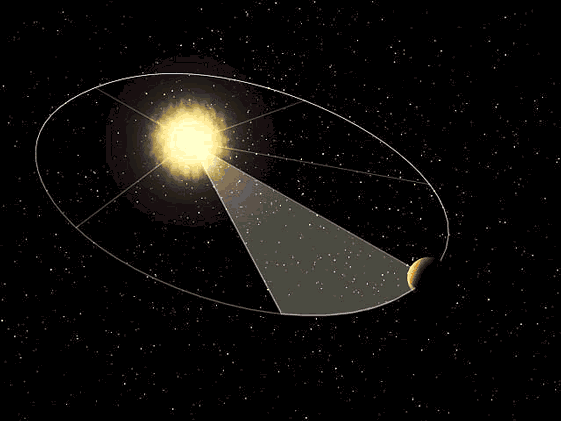
In other words, as a planet moves around the Sun in its elliptical orbit, it moves faster when it is closer to the Sun and slower when it is farther away. This means that the planet covers more area in the same amount of time when it is closer to the Sun than when it is farther away.
This law is also known as the Law of Conservation of Angular Momentum, which means that a planet’s momentum remains constant regardless of its position in its orbit.
Kepler’s Third Law: The Law of Harmonies
The third and final of Kepler’s Laws of Planetary Motion is the Law of Harmonies. This law relates the period of a planet’s orbit around the Sun to the planet’s distance from the Sun.
Specifically, this law states that the square of the period of a planet’s orbit is proportional to the cube of its average distance from the Sun. This means that the farther a planet is from the Sun, the longer it takes to complete one orbit around the Sun.
This law is essential in determining the relative distances between planets in our solar system. It also allows astronomers to make predictions about the orbits of planets around other stars.
Why Are Kepler’s Laws Important?
Kepler’s Laws of Planetary Motion are essential in our understanding of the universe. They allowed astronomers to accurately predict the position of planets in the sky at any given time. This was crucial for navigation and timekeeping.
Kepler’s Laws also paved the way for Sir Isaac Newton’s discovery of the laws of gravitation. His discovery further advanced our understanding of the motion of objects in space.
Kepler’s Laws of Planetary Motion are a fundamental part of our understanding of the cosmos. These laws have helped us to understand the motion of planets in our solar system. They have paved the way for countless scientific discoveries over the past four centuries.
By studying Kepler’s Laws, we can deepen our understanding of the universe we live in and gain a new appreciation for the beauty and complexity of the cosmos.
Return to Insolation and Heat Budget